Study of Crystals using X-rays by W.H. & W.L. Bragg
Von Laue’s epoch-making discovery of the diffraction of the X-rays in crystals, on the one hand established wave motion as the essential quality of those rays and, on the other, afforded the experimental proof of the existence of molecular gratings in the crystals. The problem, however, of calculating the crystal structures from von Laue’s formulae was an exceedingly complicated one, inasmuch as not only the space lattices, but also the wavelengths and the intensity-distribution over the various wavelengths in the spectra of the X-rays, were unknown quantities.
It was consequently a discovery of epoch-making significance when William Lawrence Bragg found out that the phenomenon could be treated mathematically as a reflection by the successive parallel planes that may be placed so as to pass through the lattice points, and that in this way the ratio between the wavelengths and the distances of the said planes from each other can be calculated by a simple formula from the angle of reflection.

It was only by means of that simplification of the mathematical method that it became possible to attack the problem of the crystal structures, but to attain the end in view it was further necessary that the photographic method employed by von Laue should be replaced by an experimental one, based on the reflection principle, which admitted of a definite, even though at first unknown, wavelength being made use of. The instrument requisite for the said purpose, the so-called X-ray spectrometer, was constructed by Professor Sir William Henry Bragg, W.L.Bragg’s father, and it has been with the aid of that instrument that father and son have carried out, in part conjointly, in part each on his own account, a series of extremely important investigations respecting the structure of crystals.
The space lattice in the regular or cubic system must therefore coincide with one of those three, or constitute combinations of them. In such lattice combinations, on the other hand, in which the condition just mentioned is not fulfilled, where consequently parallel planes placed to pass through all the lattice points in certain directions are not equidistant, that circumstance is revealed by an abnormal intensity distribution among spectra of different orders, when the reflection takes place by those planes above is a brief sketch of the methods discovered by the two Braggs for investigating crystal structures. The results of their investigations embrace a large number of crystals belonging to various systems and can only be cursorily summarized in this place.
As it must be assumed, on the strength of the analogy of these salts, both in a chemical and a crystallographical sense, that they are possessed of a corresponding space lattice, which could also be corroborated in another way, it was proved by those researchers that the lattice of the crystals in question consists of two face-centred cubic lattices corresponding to the two atoms, which interpenetrate in such a way that they together constitute one single cubic lattice.
They tried to attack the problem from a slightly different point of view, and to see what would happen if a series of irregular pulses fell on diffracting points arranged on a regular space lattice. This led naturally to the consideration of the diffraction effects as a reflexion of the pulses by the planes of the crystal structure.
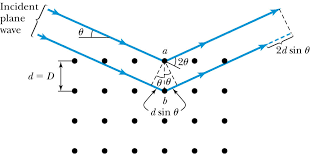
The points of a space lattice may be arranged in series of planes, parallel and equidistant from each other. As a pulse passes over each diffracting point, it scatters a wave, and if a number of points are arranged on a plane the diffracted wavelets will combine together to form a reflected wave front, according to the well-known Huygens construction. The pulses reflected by successive planes build up a wave train, which analysis shows to be composed of the wavelengths given by the formula:
nλ = 2dsin(θ)
In this expression, n is an integer, λ is the wavelength of the X-rays, d the spacing of the planes, and θ the glancing angle at which the X-rays are reflected.
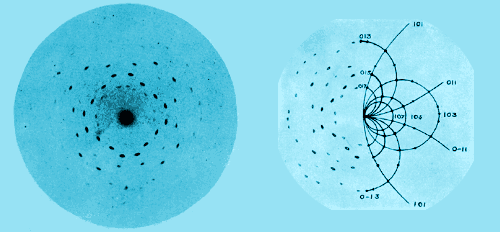
A further development of the powder method which has proved to be most interesting is the study of colloidal particles. Scherrer has shown that particles of metals in the colloidal state consist of minute crystals, with a structure like that of large crystals; he has even formed an estimate of the size of the particles by observing the broadening of the haloes, this being due to a loss of resolving power owing to the small number of reflecting planes in any one particle. There seems to be hardly any type of matter in the condition of a true solid which we cannot attempt to analyse by means of X-rays. For the first time the exact arrangement of the atoms in solids has become known; we can see how far the atoms are apart and how they are grouped. The importance of this consists in the light it throws on chemical composition.
Finally it may be mentioned that the two investigators have also determined the wavelengths of the X-rays and the distances between the successive planes placed to pass through the lattice points with such exactitude, that the error, if any, is probably at most some few units per cent and is more due to the general physical constant entering into the calculations than to the measurements themselves.
Thanks to the methods that the Braggs, father and son, have devised for investigating crystal structures, an entirely new world has been opened and has already in part been explored with marvellous exactitude. The significance of these methods, and of the results attained by their means, cannot as yet be gauged in its entirety, however imposing its dimensions already appear to be. In consideration of the great importance that these methods possess for research in the realm of physics, the Swedish Royal Academy of Sciences decided that the 1915 Nobel Prize in Physics should be divided between Professor W. H. Bragg and his son W. L. Bragg, in recognition of their services in promoting the investigation of crystal structures by means of X-rays.
Reflection of X-rays by Crystals (I.)Reflection of X-rays by Crystals (II.)WILLIAM LAWRENCE BRAGG - Nobel Lecture

REFERENCES
Florida State University. Available in: http://www.hep.fsu.edu/~wahl/NobLect/WHBraggWLBragg1915.pdf. Access in: 11/10/2018.
Encyclopaedia Britannica. Available in: https://www.britannica.com/science/Bragg-law. Access in: 11/10/2018.
The Royal Society. Available in: http://rspa.royalsocietypublishing.org/content/88/605/428. Access in: 11/10/2018.












































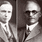






































0 comments
Sign in or create a free account